When it comes to comparing vehicle specifications, especially between different classes like a Lamborghini Truck and a traditional Ford truck, the numbers can be overwhelming and often used more for marketing than clear understanding. Deciphering horsepower, torque, and acceleration figures requires a deeper dive into the mechanics at play. Let’s break down the key factors that influence vehicle performance using the Lamborghini Urus (often referred to as a Lamborghini truck due to its SUV form) and a Ford truck as contrasting examples.
The fundamental principle governing a vehicle’s acceleration ($a$) is Newton’s second law: Force ($F$) equals mass ($m$) times acceleration ($a$), or $F = ma$. This means acceleration is directly proportional to the force applied to the vehicle and inversely proportional to its mass. The power ($P$) and torque ($tau$) specifications provided by manufacturers relate to the engine’s capabilities at specific engine speeds ($omega$), linked by the equation $P = tau omega$.
To illustrate the differences, consider the power and torque curves of a Lamborghini Urus engine compared to a Ford truck engine. While specific models and years can vary, the general characteristics are depicted in the graph below, redrawn for clarity and consistent units:
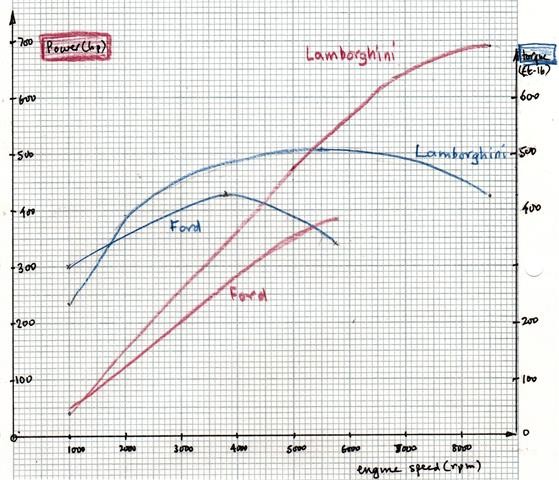Lamborghini Urus vs Ford Truck Engine Power and Torque Curves
As you can observe, the Lamborghini engine is designed to reach higher maximum engine speeds. Crucially, the mass difference between these vehicles significantly impacts acceleration. The Lamborghini Urus, weighing in at approximately 1690 kg (3725 lbs), is considerably lighter than a Ford truck, which can be around 2745 kg (6052 lbs) or more. This weight disparity means that for the same amount of force applied, the lighter Lamborghini will achieve much greater acceleration.
However, engine torque is not directly delivered to the wheels. It’s modified through the drivetrain, starting with gear ratios in the transmission. Gear ratios reduce engine speed and proportionally increase torque. To maximize torque at the wheels, lower gears (higher gear ratios) are utilized.
Let’s examine the gear ratios of both vehicles. The Lamborghini Urus typically features 7 forward gears with ratios like:
1st: 3.91:1 / 2nd: 2.44:1 / 3rd: 1.81:1 / 4th: 1.46:1 / 5th: 1.19:1 / 6th: 0.97:1 / 7th: 0.84:1
Combined with a differential ratio of 3.54:1, the total torque multiplication in first gear is approximately 3.91 * 3.54 ≈ 13.8.
Ford trucks, depending on the model, might have 6 or more gears. As an example, a Ford truck could have gear ratios around:
1st: 3.97:1 / 2nd: 2.31:1 / 3rd: 1.51:1 / 4th: 1.14:1 / 5th: 0.85:1 / 6th: 0.67:1
With a differential ratio of 3.73:1, the torque multiplication in first gear is approximately 3.97 * 3.73 ≈ 14.8, slightly higher than the Lamborghini in this simplified comparison. Certain Ford truck versions, especially turbo-diesel models, boast even higher torque figures and gear ratios, further increasing torque at the wheels, particularly in lower gears, which is beneficial for tasks like towing and hauling.
Another factor influencing the force at the wheels is the wheel radius. The relationship is:
Torque at wheels = Accelerating force × Radius of wheels
The Lamborghini Urus, being a performance SUV with four-wheel drive, may have front tires around 335/30YR20 and rear tires around 255/35YR19, translating to approximate radii of 14 inches for the front and 13 inches for the rear. Ford trucks, designed for utility, often have larger wheel radii. For instance, a Ford truck with 265/75R16 tires might have a radius of about 15.8 inches. A larger wheel radius means that for the same torque at the wheels, the accelerating force is reduced.
Considering all these factors, the Lamborghini Urus generally achieves superior acceleration primarily because of its significantly lower mass. While Ford trucks can deliver substantial torque, especially at lower speeds and in lower gears, optimized for work and towing, the weight and gearing are different.
Once vehicles are in motion, aerodynamic drag becomes a crucial factor, especially at higher speeds. Drag force is proportional to the square of velocity and is given by $F_{rm drag} = frac{1}{2} c_D A rho v^2$, where $c_D$ is the drag coefficient, $A$ is the frontal area, $rho$ is air density, and $v$ is velocity.
Lamborghini Urus is designed with a lower drag coefficient (around 0.33) and a smaller frontal area (approximately $2.3 , rm m^2$) compared to a Ford truck (drag coefficient around 0.4 or higher and a larger frontal area, perhaps $8 , rm m^2$). These aerodynamic advantages, combined with a powerful engine, enable the Lamborghini Urus to reach a much higher top speed, around 190 mph (305 km/h), while a Ford truck, focused on utility, will have a considerably lower top speed.
In essence, the Lamborghini truck (Urus) and Ford truck are engineered for different purposes. Ford trucks prioritize torque for heavy loads and work applications, utilizing gearing to amplify engine torque, while Lamborghini focuses on speed and agility, leveraging lower mass, higher engine speeds, and aerodynamic efficiency. The Lamborghini’s engine design philosophy prioritizes increasing power through higher engine speeds, whereas truck engines often prioritize robust torque at lower RPMs for work-related tasks.
